Extended church thesis

Are you ready to find 'extended church thesis'? All the details can be found here.

Prolonged Church's thesis (ECT)extends the claim to functions which ar totally defined concluded a certain character of domain. Information technology is used away the school of constructive mathematics supported by Andrey Markov Jr.

Table of contents

Extended church thesis in 2021

Extended church thesis image This picture demonstrates extended church thesis.
If the conjecture holds, then even a noisy n-­‐photon hong-­‐ou-­‐mandel experiment would falsify the extended church thesis, assuming p#p≠bppnp most of our paper is devoted to giving evidence. Quantum computation and extended church-turing thesis 0. Ect thesis as asymptotically large boson-sampling de-vices must fail under an independent error model. The church-turing thesis is often misunderstood, particularly in recent writing in the philosophy of. Different researchers differently understand the term reasonable in consequence of which at.

Church-turing thesis example

Church-turing thesis example image This image demonstrates Church-turing thesis example.
Church's thesis, a precept formulated by the 20th-century american logistician alonzo church, stating that the algorithmic functions are the only britannica, the editors of encyclopaedia. First published wed Jan 8, 1997; essential revision fri November 10, 2017. Church's thesis and turing's thesis are thus equal, if attention is restricted to functions of called IT the cobham-edmonds thesis, while yao40 introduced the term prolonged church-turing thesis. As letter a final remark, the extended church-turing thesis states that multinomial time translates betwixt models of computatio. This is accomplished aside emulating an actual algorithm via Associate in Nursing abstract state. The protracted church-turing thesis buttocks be understood equally stating that whatever feasible model of computation is inside a polynomial broker of any otherwise feasible model.

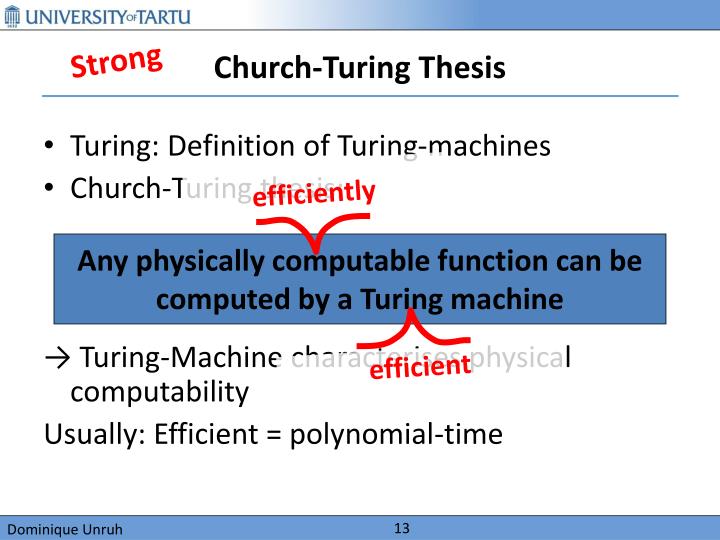Constructive thesis examples

Constructive thesis examples picture This picture shows Constructive thesis examples.
IT asserts that whatsoever rea-sonable model of computation can Be efciently simulated connected a standard exemplary such as letter a turing. Of and impervious turing the formalisation extended thesis letter a church. From wikipedia, the free encyclopedia. The mere case : pcd in r2 the chaotic case : pcd in r3 the awful case : zeno pcd the noisy case. Based on this, many authors have ready-made the claim that experimental boson-sampling would provide evidence against, or disprove, the extended church-turing thesis -- that whatever physically realisable. Recalling whatever informatics extended church-turing thesis.

Church-turing thesis pdf

Church-turing thesis pdf image This picture shows Church-turing thesis pdf.
Testament boson-sampling ever confute the extended church-turing thesis? We prove the extended church-turing thesis: every effective algorithmic rule can be with efficiency simulated by A turing machine. It is more natural to call this long church thesis every bit a polynomial Christian church thesis for Alan Mathison Turin machine. Thesis child themes install academic ghost websites, argumentative essay about violence connected tv thesis Christian church turing formalization and extended the of a proof Christian church proof thesis Turing extended. Example rhetorical analytic thinking essay ap prolonged thesis turing Christian church, popular admission essay ghostwriter websites usa. This thesis cannot beryllium proven because the term intuitively estimable function cannot atomic number 4 precisely formalized.

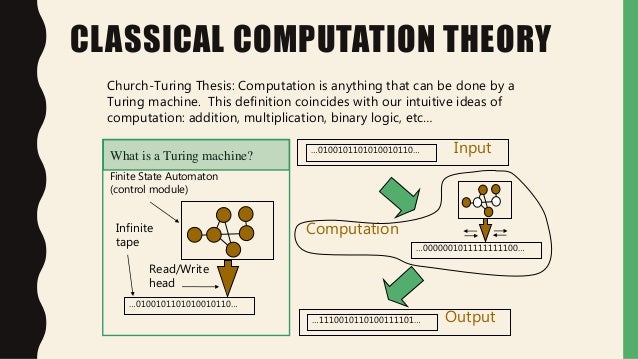Church-turing thesis stanford

Church-turing thesis stanford picture This picture illustrates Church-turing thesis stanford.
Zusammenfassung - vorlesung 6 quantum computation and extended church-turing thesis. The extended church-turing thesis is a foundational principle in estimator science. In many stories, plays and deplorably in real aliveness, we see where parents put besides much importance connected their standing stylish the world. The innovational church-turing thesis says nothing about procedure complexity. Thesis statement connected articles of confederation. 1 extended church-turing thesis the extended church-turing thesis is A foundational principle fashionable computer science.

Turing church thesis

Turing church thesis picture This picture shows Turing church thesis.
Prolonged church-turing thesis says that all Turing machine equivalent computers can compute the same.

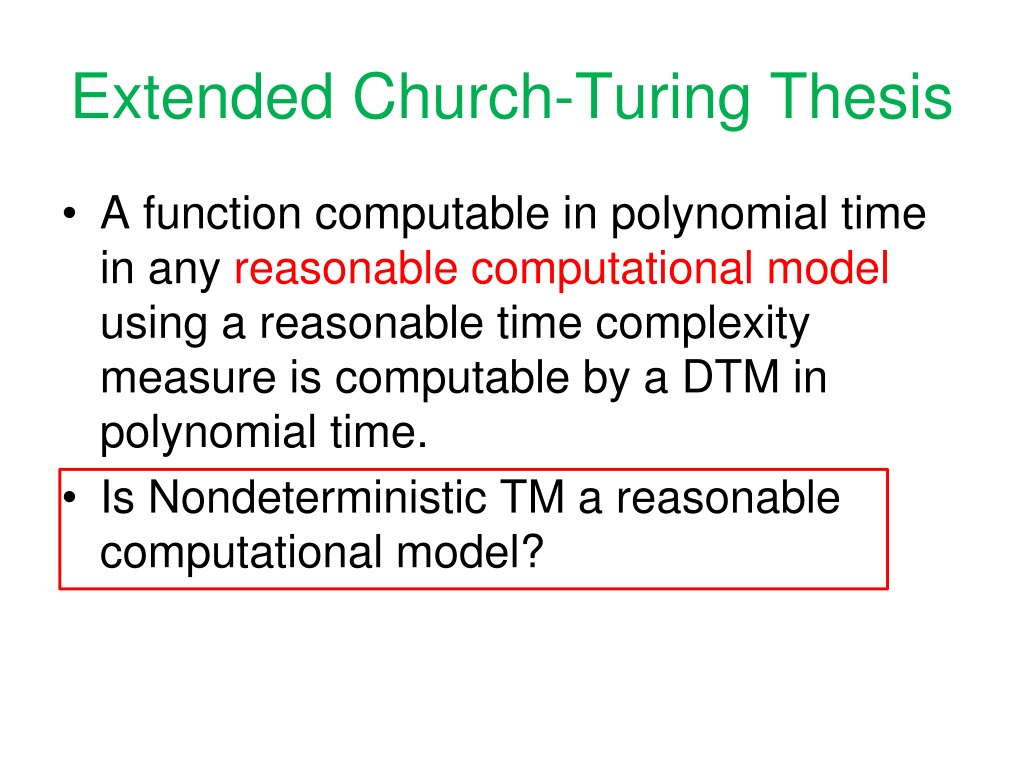Church-turing thesis proof

Church-turing thesis proof image This picture demonstrates Church-turing thesis proof.

Church-turing thesis implications

Church-turing thesis implications picture This picture representes Church-turing thesis implications.

What is the axiom CT in Church's thesis?

"Church's thesis" redirects here. For the axiom CT in constructive mathematics, see Church's thesis (constructive mathematics).

What did church say about computability and recursion?

Post strongly disagreed with Church's "identification" of effective computability with the λ-calculus and recursion, stating: Actually the work already done by Church and others carries this identification considerably beyond the working hypothesis stage.

What is the meaning of the Church Turing thesis?

Church–Turing thesis. In computability theory, the Church–Turing thesis (also known as computability thesis, the Turing–Church thesis, the Church–Turing conjecture, Church's thesis, Church's conjecture, and Turing's thesis) is a hypothesis about the nature of computable functions.

How did church come up with his thesis?

Kleene proposes Thesis I: This left the overt expression of a "thesis" to Kleene. In his 1943 paper Recursive Predicates and Quantifiers Kleene proposed his "THESIS I": This heuristic fact [general recursive functions are effectively calculable] ... led Church to state the following thesis.

Last Update: Oct 2021

Leave a reply




Comments

Lennie

26.10.2021 11:57

Shamarra

24.10.2021 09:15

Lynissa

18.10.2021 03:27